Question

The graph shows the relationship between the number of hours a kayak is rented and the total cost of the rental. Write an equation of the relationship then use the equation to predict the cost of a rental last 5.5 hours

Answer 1

17

Explanation:

im insane

Answer 2

The equation representing the relationship between the number of hours a kayak is rented
`(\(x\))` and the total cost of the rental
`(\(y\))` can be written as
`\(y = mx + b\), where \(m\)` is the slope and
`\(b\)` is the y-intercept. To find these values, refer to the graph or given data. Once the equation is established, use it to predict the cost of a rental lasting 5.5 hours.

Explanation:

To derive the equation, analyze the graph or data provided. Identify two points on the graph, which can serve as coordinates
`\((x_1, y_1)\) and \((x_2, y_2)\)`. The slope
`(\(m\))` is calculated as the change in
`\(y\)` divided by the change in
`\(x\), i.e., \(m = (y_2 - y_1)/(x_2 - x_1)\).` The y-intercep
`t (\(b\))` is the value of
`\(y\) when \(x = 0\).`Substitute the values o f
`\(m\) and \(b\)` into the equation
`\(y = mx + b\).`

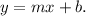
For instance, if the slope
`(\(m\))` is found to be 8 and the y-intercept
`(\(b\))` is 20, the equation becomes
`\(y = 8x + 20\).` This equation represents the relationship between the number of hours a kayak is rented
`(\(x\))` and the total cost of the rental
`(\(y\)).`

To predict the cost of a rental lasting 5.5 hours, substitute \(x = 5.5\) into the equation:

`\[ y = 8 * 5.5 + 20 \]`

Calculate the result to find the predicted cost for a rental of 5.5 hours.

In conclusion, by establishing the equation representing the relationship between hours rented and total cost, and subsequently using this equation to predict the cost for a given duration, one can make informed decisions based on the provided data or graph. This process emphasizes the application of mathematical concepts in real-world scenarios, enabling practical predictions and analyses.