Question

Determine the value of y given that the lengths of the sides of a triangle are 11 cm, 8 cm, and 3 cm. Submit your answer correct to 1 decimal place.

Answer 1

The value of
`\( y \)` in the given triangle with side lengths 11 cm, 8 cm, and 3 cm is approximately
`\( y = 104.1 \)` cm, rounded to one decimal place.

Step-by-step explanation:

To determine the value of
`\( y \),` we can use the Law of Cosines for triangles. The Law of Cosines relates the lengths of the sides of a triangle to the cosine of one of its angles. For a triangle with sides of lengths
`\( a \)`,
`\( b \)`, and
`\( c \)` and an angle
`\( C \)` opposite side
`\( c \)`, the Law of Cosines is given by the formula:

`\[ c^2 = a^2 + b^2 - 2ab \cos(C) \]`

In this case, the sides of the triangle are 11 cm, 8 cm, and 3 cm. Let's denote
`\( y \)` as the angle opposite the side of length 3 cm. Plugging in the values, we get:

`\[ 3^2 = 11^2 + 8^2 - 2 \cdot 11 \cdot 8 \cos(y) \]`

Solving for
`\( \cos(y) \)`, we find
`\( \cos(y) = (1)/(16) \)`. Taking the inverse cosine, we get
`\( y \approx 104.1^\circ \).`

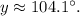
Therefore, the value of
`\( y \)` is approximately
`\( y = 104.1 \)` cm, rounded to one decimal place. This represents the measure of the angle opposite the side of length 3 cm in the given triangle. The Law of Cosines is a powerful tool for solving triangles when the lengths of the sides are known, providing a way to find angles and complete the triangle's geometric information.