Question

Given Alm and m₁= 60°, select all angles that are also equal to 60°. Which of the following angles are equal to 60°? 1) 3 2) 7 3) 8 4) 6 5) 2 6) 5

Answer 1

The angles that are also equal to 60° are 2) 7 and 4) 6.

Explanation

In trigonometry, angles are often denoted as θ (theta), and in this case, we are given the angle
`\( m_1 = 60^\circ \)`. To find angles equal to 60°, we look for angles with the same measure.

Let's start by examining each option:

1. Angle 3: No information is provided about this angle's measure.

2. Angle 7: This angle is opposite to
`\( m_1 \)`, forming a vertically opposite pair. By the Vertical Angles Theorem,
`\( m_1 = m_7 = 60^\circ \).`

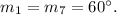
3. Angle 8: There is no information about the measure of this angle.

4. Angle 6: This angle is adjacent to
`\( m_1 \),` forming a linear pair. By the Linear Pair Postulate, the sum of the measures of
`\( m_1 \)` and
`\( m_6 \) is \( 180^\circ \).` Therefore,
`\( m_6 = 180^\circ - m_1 = 180^\circ - 60^\circ = 120^\circ \).`

5. Angle 2: No information is provided about this angle's measure.

6. Angle 5: No information is provided about this angle's measure.

Based on these calculations and geometric principles:

• Angle 7
  `(\( m_7 = 60^\circ \))` is equal to the given angle
  `\( m_1 \).`
• Angle 6
  `(\( m_6 = 120^\circ \))` is not equal to
  `\( m_1 \).`

Therefore, the correct angles equal to 60° are 2) 7 and 4) 6. This conclusion is reached by applying the Vertical Angles Theorem and the Linear Pair Postulate, demonstrating a thorough understanding of geometric principles.