Question

write the equation of the quadratic function in standard form that is represented by the graph.. (2,0) (4,-3) (10,0)

Answer 1

so the quadratic has roots or x-intercepts at 2 and 10, or namely it has zeros when x = 2 and x = 10, whilst it passes through (4 , -3).

Now, let's reword all that

what's the equation of a quadratic whose roots are 2 and 10 and it passes through (4 , -3)?

`\begin{cases} x = 2 &\implies x -2=0\\ x = 10 &\implies x -10=0 \end{cases} \\\\[-0.35em] ~\dotfill\\\\ \stackrel{original~polynomial}{a ( x -2 )( x -10 ) = \stackrel{0}{y}} \hspace{5em}\textit{we also know that } \begin{cases} x=4\\ y=-3 \end{cases} \\\\\\ a ( 4 -2 )( 4 -10 ) = -3\implies a(2)(-6)=-3\implies -12a=-3 \\\\\\ a=\cfrac{-3}{-12}\implies a=\cfrac{1}{4} \\\\[-0.35em] ~\dotfill`

`\cfrac{1}{4}(x-2)(x-10)=y\implies \cfrac{1}{4}(x^2-12x+20)=y \\\\\\ ~\hfill~ {\Large \begin{array}{llll} \cfrac{1}{4}x^2-3x+5=y \end{array}}~\hfill~`

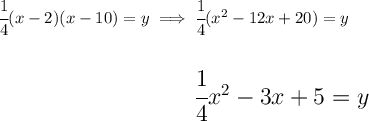
Check the picture below.