Question

7. (1+1+2+2+1+1=8 marks ) An engineer of a company wanted to obtain the information about the power failures in a small town. He recorded the number of power failures per day for the period of 50 consecutive days. He has found that there were 4 days with no failures, 15 days with 1 failure, 16 days with 2 failures, and 15 days with 3 or more failures. The data obtained by the engineer are given in the table below. Test at the 0.05 level of significance whether the number of power failures in the town per day is a random variable having Poisson distribution with the value of parameter λ estimated from the sample data. Follow the steps: a. Use sample data to show that estimated mean of Poisson distribution is λ=1.84. (1 mark) b. State the hypotheses and the level of significance. (1 mark) H 0​ : H 1 : c. Calculate the expected frequencies using appropriate row for each calculation. In the expressions for expected frequencies leave two digits after decimal point. (2 marks). Place calculate expected frequencies into the last row of the table on the top of the page 8 . Combine the cells with expected frequencies smaller than 5 (only if required). d. Calculate the value of test statistic using sample data and calculated expected frequencies. (2 marks) χ obs.2​ = e. Determine critical value of the test. (1 mark) χ cr2​ . f. Determine whether H 0 ​ is rejected and make the conclusions whether the number of power failures in the town per day is a random variable having Poisson distribution with the value of parameter λ=1.84. ( 1 mark)

Answer 1

a. The estimated mean
`(\( \lambda \))` of the Poisson distribution, based on the sample data, is calculated to be
`\( \lambda = 1.84 \).`

b. The hypotheses are:

`\[ H_0: \text{The number of power failures follows a Poisson distribution with } \lambda = 1.84 \]`

`\[ H_1: \text{The number of power failures does not follow a Poisson distribution with } \lambda = 1.84 \]`

c. The expected frequencies for each category are calculated using the Poisson distribution formula, and these values are placed in the last row of the table. If any expected frequency is less than 5, the corresponding cells are combined.

d. The test statistic
`(\( \chi_{\text{obs.2}} \))` is calculated using the sample data and the expected frequencies.

e. The critical value of the test
`(\( \chi_{\text{cr.2}} \))` is determined.

f. Comparing the calculated test statistic to the critical value, if
`\( \chi_{\text{obs.2}} > \chi_{\text{cr.2}} \)`, we reject
`\( H_0 \)`. In this case, we would conclude that the number of power failures per day does not follow a Poisson distribution with
`\( \lambda = 1.84 \).`

Step-by-step explanation:

a. The estimated mean of the Poisson distribution
`(\( \lambda \))` is calculated from the sample data using the formula
`\( \lambda = \frac{\sum{(f_i \cdot x_i)}}{n} \),` where
`\( f_i \)` is the frequency and
`\( x_i \)` is the number of power failures in each category.

b. The null hypothesis
`(\( H_0 \))` assumes that the observed data follows a Poisson distribution with
`\( \lambda = 1.84 \)`, while the alternative hypothesis
`(\( H_1 \))` suggests otherwise.

c. Expected frequencies are calculated using the Poisson distribution formula:
`\( E_i = (e^(-\lambda) \cdot \lambda^(x_i))/(x_i!) \)`. These values are then placed in the last row of the table. If any expected frequency is less than 5, the corresponding cells are combined to meet the assumption of the chi-square test.

d. The test statistic
`\( \chi_{\text{obs.2}} \)` is calculated using the formula:
`\( \chi_{\text{obs.2}} = \sum{((O_i - E_i)^2)/(E_i)} \)`, where
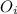`\( O_i \)` is the observed frequency and
`\( E_i \)` is the expected frequency.

e. The critical value
`\( \chi_{\text{cr.2}} \)` is determined based on the degrees of freedom and the significance level.

f. By comparing the calculated test statistic to the critical value, a decision is made on whether to reject the null hypothesis. If
`\( \chi_{\text{obs.2}} > \chi_{\text{cr.2}} \),` we reject
`\( H_0 \)`, indicating that the number of power failures per day does not follow a Poisson distribution with
`\( \lambda = 1.84 \).`