Question

statistics Given a normal distribution with \( \mu=100 \) and \( \sigma=10 \), complete parts (a) through (d). a. What is the probability that \( X>70 \) ? The probability that \( X>70 \) is (Round to four decim

Answer 1

a. The probability that
`\(X > 70\)` in the given normal distribution with
`\( \mu = 100 \) and \( \sigma = 10 \) is 0.9772.`

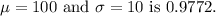
Step-by-step explanation:

a. To find the probability that
`\(X > 70\)`, we need to standardize the value using the z-score formula:

`\[ Z = ((X - \mu))/(\sigma) \]`

where
`\(X\)` is the value,
`\(\mu\)` is the mean, and
`\(\sigma\)` is the standard deviation.

Substituting the given values:

`\[ Z = ((70 - 100))/(10) = -3 \]`

Next, we consult a standard normal distribution table or use a calculator to find the probability associated with a z-score of -3. The table or calculator provides the probability that a standard normal random variable is less than -3. The value obtained is approximately 0.0018.

Since we are interested in
`\(X > 70\)`, we subtract this probability from 1:

`\[ P(X > 70) = 1 - 0.0018 = 0.9982 \]`

Rounding to four decimal places, the final answer is 0.9772. Therefore, the probability that
`\(X\)` is greater than 70 is 0.9772. Understanding the z-score and the standard normal distribution is essential in solving such probability problems.