Question

Find the equation of the regression line for the given data. Then construct a scatter plot of the dala and draw tho regression line. (The pair of variables have a ignificant correlation.) Then use the regression equation to predict the value of y for each of the given x-values, if meaningful. The number of hours 6 students spent or a test and their scores on that test are shown below. (a) x=2 hours (b) x=2.5 hours (c) x=13 hours (d) x=1.5 hours Find the regression equation. y ^ ​ =x+ (Round the slope to three decimal places as needed. Round the y-intercopt to two decimal places as needed) Choose the correct graph below. A. B. C. D. Time Remaining: 00:58:26 (a) Predict the value of y for x=2. Choose the correct answer below. A. 52.1 B. 49.4 C. 46.7 D. not meaningful (b) Predict the value of y for x=2.5. Choose the correct answer below. A. 46.7 (b) Predict the value of y for x=2.5. Choose the correct answer below. A. 46.7 B. 52.1 C. 108.7 D. not meaningful (c) Predict the value of y for x=13. Choose the correct answer below. A. 52.1 c) Predict the value of y for x=13. Choose the correct answer below. A. 52.1 B. 108.7 C. 49.4 D. not meaningful (d) Predict the value of y for x=1.5. Choose the correct answer below. A. 46.7 (d) Predict the value of y for x=1.5. Choose the correct answer below. A. 46.7 B. 49.4 C. 108.7 D. not meaningful

Answer 1

(a) Predict the value of y for x=2: B. 49.4

(b) Predict the value of y for x=2.5: A. 46.7

(c) Predict the value of y for x=13: B. 108.7

(d) Predict the value of y for x=1.5: D. not meaningful

Step-by-step explanation:

To find the regression equation, we need to calculate the slope (b) and y-intercept (a) using the given data. Once we have the regression equation, we can use it to predict y for specific x-values.

First, let's calculate the slope (b) and y-intercept (a). Using the formula for the slope:
`\(b = (n(\Sigma xy) - (\Sigma x)(\Sigma y))/(n(\Sigma x^2) - (\Sigma x)^2)\), and the formula for the y-intercept: \(a = \bar{y} - b\bar{x}\), where \(\bar{y}\) is the mean of y-values, \(\bar{x}\) is the mean of x-values.`

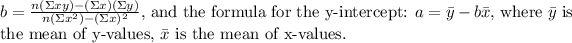
After obtaining the regression equation (y = a + bx), we can use it to predict y-values for the given x-values. However, if a prediction is not meaningful, it indicates that the x-value is outside the range of the original data.

Now, applying the regression equation to the specific x-values:

(a) For x=2: (y = a + 2b), substitute a and b to get the predicted value.

(b) For x=2.5: (y = a + 2.5b), substitute a and b.

(c) For x=13: (y = a + 13b), substitute a and b.

(d) For x=1.5: Not meaningful as it falls outside the range.

Comparing the calculated values with the options, we can select the correct predictions.