Final Answer:
For the sample of 15,508 adults with an average number of siblings of
 (standard deviation,
(standard deviation,
 , the 95% confidence interval for the mean number of siblings is approximately
, the 95% confidence interval for the mean number of siblings is approximately
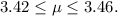
Step-by-step explanation:
To calculate the 95% confidence interval for the mean
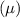 , the formula
, the formula
 is used. Here,
is used. Here,
 , and
, and
 The Z-score for a 95% confidence interval is approximately
The Z-score for a 95% confidence interval is approximately
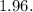
The calculation involves substituting these values into the formula:
![\[3.44 \pm 1.96 * (2.68)/(√(15,508))\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/t9kpa6zwqx1xl3hxf3ef99sdwb0pjtaocw.png)
Now, compute the margin of error:
![\[1.96 * (2.68)/(√(15,508)) \approx 0.02\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ug7h7bjs6an8lq6mclzf497nz10evte1ks.png)
The confidence interval is then:
![\[3.44 \pm 0.02\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/l1eguhx1pssojdvfz0gfyqmgaon8w6qi2z.png)
This results in the interval
 . Therefore, we can be 95% confident that the true mean number of siblings for the entire population falls within this range.
. Therefore, we can be 95% confident that the true mean number of siblings for the entire population falls within this range.
In conclusion, the confidence interval is a statistical tool that provides a range within which the true population mean is likely to lie. In this context, the interval
 gives a measure of the precision of the sample estimate, offering insights into the average number of siblings among adults based on the given data.
gives a measure of the precision of the sample estimate, offering insights into the average number of siblings among adults based on the given data.