Question

f. What is the probability that the sampling error ( xˉ −μ) would be $9 or more? That is, what is the probability that the estimate of the population mean is less than $51 or more than $69 ? (Round z-value to 2 decimal places and final answer to 4 decimal places.)

Answer 1

The probability that the sampling error would be $9 or more (estimate of the population mean is less than $51 or more than $69) is approximately 0.0244.

Step-by-step explanation:

Given:

Population mean (μ) = $60

Population standard deviation σ = $12

Sample size (n) = 9

To find this probability, we first compute the z-scores for both $51 and $69 using the formula:

`\[ z = (x - \mu)/((\sigma)/(√(n))) \]`

Given the mean
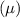`(\(\mu\))` is $60, standard deviation
`(\(\sigma\))` is $12, sample size n is 9, the z-score for $51 and $69 is calculated as follows:

For $51:

z = {51 - 60}/(12/√9)} = -9/4 = -2.25

For $69:

z = {69 - 60}/(12/√9) = 9/4 = 2.25

By consulting a standard normal distribution table or using a calculator that provides cumulative probabilities for the standard normal distribution, we find:

The area to the left of -2.25 is approximately 0.0122.

The area to the right of 2.25 is also approximately 0.0122.

Therefore, the probability that the sampling error would be $9 or more (estimate of the population mean is less than $51 or more than $69) is the combined area of both tails, which is approximately

0.0122+0.0122=0.0244.

Rounded to four decimal places, the final probability is approximately 0.0244, representing the probability that the sampling error would be $9 or more.

Complete Question

A normal population has a mean of $60 and standard deviation of $12. You select random samples of nine.

What is the probability that the sampling error ( x⎯⎯x¯ − μ) would be $9 or more? That is, what is the probability that the estimate of the population mean is less than $51 or more than $69? (Round z value to 2 decimal places and final answer to 4 decimal places.)