Question

Hi, can you help me answer this question please, thank you!

Answer 1

Given:

Two populations

Sample Size (n₁) = 202

Success (x₁) = 122

Sample size (n₂) = 340

Success (x₂) = 220

Find: test statistic and p-value of this sample

Solution:

Based on the given data, we have two proportions here and its sample size is large. The test statistic that is appropriate for this would be Test of Two Proportions and the formula is:

`z=\frac{p_1-p_2+cont\text{ }}{\sqrt[]{(p(1-p))/(n_1)+\frac{p(1-p)_{}}{n_2}}}`

in which,

`p=(x_1+x_2)/(n_1+n_2)`

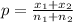
Let's solve the value of p first. Let's plug in the given data that we have above.

`p=(122+220)/(202+340)=(342)/(542)=(171)/(271)`

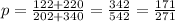
Now that we have the value of p, let's calculate p₁ and p₂. Formula is:

`\begin{gathered} p_1=(x_1)/(n_1)=(122)/(202)=(61)/(101) \\ p_2=(x_2)/(n_2)=(220)/(340)=(11)/(17) \end{gathered}`

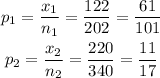
Lastly, let's calculate the value of cont or continuity correction. Formula is:

`cont=(F)/(2)((1)/(n_1)+(1)/(n_2))\text{ }`

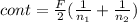
For our claim p₁ < p₂, our F = 1.

`cont=(1)/(2)((1)/(202)+(1)/(340))=0.0039458`

Let's plug these values to the test of two proportions formula:

`\begin{gathered} z=\frac{p_1-p_2+cont\text{ }}{\sqrt[]{(p(1-p))/(n_1)+\frac{p(1-p)_{}}{n_2}}} \\ z=\frac{(61)/(101)-(11)/(17)+0.0039458}{\sqrt[]{((171)/(271)(1-(171)/(271)))/(202)+\frac{(171)/(271)(1-(171)/(271))_{}}{340}}} \end{gathered}`
`z=\frac{-0.03915259}{\sqrt[]{(0.2328399668)/(202)+(0.2328399668)/(340)}}=(-0.03915259)/(0.04286603008)\approx-0.913`

Hence, the test statistic is -0.913.

The equivalent p-value for this is 0.1805.

The p-value is greater than α = 0.05.

Since p-value is greater than α, we fail to reject the null hypothesis.