Question

You have $5,000 to invest and want it to grow to $20,000 in two years. What interest rate would you need to find to make this possible?I wan answer and explanation.

Answer 1

To grow $5,000 to $20,000 in two years, the necessary annual compound interest rate would be 100%.

Step-by-step explanation:

To determine the necessary interest rate required to grow $5,000 to $20,000 in two years, one would use the formula for compound interest, which is A = P(1 + r/n)^(nt), where:

• A is the amount of money accumulated after n years, including interest.
• P is the principal amount (the initial amount of money).
• r is the annual interest rate (decimal).
• n is the number of times that interest is compounded per year.
• t is the number of years the money is invested for.

If the interest is compounded annually (n=1), the formula simplifies to A = P(1 + r)^t. Plugging in the values gives us 20,000 = 5,000(1 + r)^2. Solving for r, we get:

4 = (1 + r)^2

2 = 1 + r

r = 1 or 100%

Thus, an annual compound interest rate of 100% would be required to quadruple the investment in two years.

Answer 2

The interest rate is 150%

Step-by-step explanation:

Given that;

The initial amount is $5000

The total amount $20, 000 after 2 years

Total period of the investment is 2 years

To find the interest rate, follow the steps below

1. Find the interest on the investment after two years

In the given data,

The initial amount (principal) is $5000

The total amount after 2 years is $20, 000

Recall that,

Total amount = Interest + principal

20, 000 = interest + 5000

subtract 5000 from both sides of the equation

20, 000 - 5,000 = interest + 5000 - 5000

15,000 = interest

Therefore, the interest on the investment after 2 years is $15, 000

Step 2; Find the interest rate using the simple interest formula

`\text{ I }=\text{ }(P* R* T)/(100)`

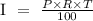
Where

I is the interest

P is the principal

R is the interest rate

T is the time of the investment

`\begin{gathered} \text{ 15, 000 }=\text{ }\frac{5000*\text{ r}*\text{ 2}}{100} \\ \text{ } \\ \text{ 15000 }=\text{ }(10,000r)/(100) \\ \text{ 15, 000 }=\text{ 100r} \\ \text{ Divide both sides by 100} \\ (15,000)/(100)\text{ }=\text{ }(100r)/(100) \\ \text{ r }=\text{ 150\%} \end{gathered}`

Therefore, the interest rate is 150%