Question

a trough shaped as half of a circular cylinder is being constructed as shown. it is able to hold cubic meters of water. additionally, its width, , is centimeters . approximating as , what is the length of the trough, , to the nearest tenth of a meter?

Answer 1

The length
`\( l \)` of the trough, to the nearest tenth of a meter, is 5.1 meters. So, the correct answer is:

C. 5.1

The trough is essentially half of a cylindrical shape, and the volume of a full cylinder is given by:

`\[ \text{Volume} = \pi r^2 h \]`

For half of a cylinder (the trough), the volume would be:

`\[ \text{Volume} = (1)/(2) \pi r^2 h \]`

where
`\( r \)` is the radius and
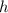`\( h \)` is the height or length of the cylinder. In this case, since the trough is lying on its side, \( h \) would be the length
`\( l \)` that we are trying to find.

Given that the volume is 0.5 cubic meters and
`\( w \)`, the diameter of the full cylinder, is 50 centimeters (or 0.5 meters since 100 centimeters make 1 meter), we can calculate the radius
`\( r \)` of the full cylinder as half of
`\( w \)`:

`\[ r = (w)/(2) = (0.5)/(2) = 0.25 \text{ meters} \]`

Substituting the values into the volume formula and solving for \( l \):

`\[ 0.5 = (1)/(2) \pi (0.25)^2 l \]`

Now let's solve for
`\( l \)` step by step:

1. Simplify the radius squared:

`\[ (0.25)^2 = 0.0625 \]`

2. Substitute into the equation:

`\[ 0.5 = (1)/(2) \pi \cdot 0.0625 \cdot l \]`

3. Multiply both sides by 2 to get rid of the fraction:

`\[ 1 = \pi \cdot 0.0625 \cdot l \]`

4. Approximating
`\( \pi \)` as 3.14:

`\[ 1 = 3.14 \cdot 0.0625 \cdot l \]`

5. Calculate
`\( 3.14 \cdot 0.0625 \)`:

`\[ 3.14 \cdot 0.0625 = 0.19625 \]`

6. Divide both sides by 0.19625 to solve for
`\( l \)`:

`\[ l = (1)/(0.19625) \]`

7. Calculate the value of
`\( l \)`:

`\[ l \approx (1)/(0.19625) \]`

The length
`\( l \)` of the trough, to the nearest tenth of a meter, is 5.1 meters. So, the correct answer is:

C. 5.1

the complete Question is given below: