Answer:
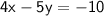
Explanation:
The standard form of a linear equation is usually written as:
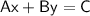
where
 ,
,
 , and
, and
 are integers, and
are integers, and
 is positive.
is positive.
The given equation is
 .
.
To write it in standard form, we can move all terms to one side of the equation and ensure that the coefficients
 ,
,
 , and
, and
 are integers:
are integers:
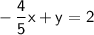
To eliminate the fraction, multiply through by 5 to clear the fraction:
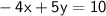
Now, to make the coefficient of
 positive, multiply through by -1:
positive, multiply through by -1:
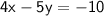
So, the equation
 written in standard form is
written in standard form is
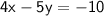 .
.