Question

Please help I cannot figure this out, different ai give me different answers

Answer 1

The sum indicated by the notation
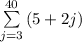`\sum\limits^(40)_(j=3) {(5 + 2j)}` is 1824

How to determine the sum indicated by the notation

From the question, we have the following parameters that can be used in our computation:

`\sum\limits^(40)_(j=3) {(5 + 2j)}`

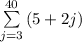
The above sigma notation is an arithmetic sequence that can be represented as

f(j) = 5 + 2j

Using the nth term of an arithmetic sequence, we have

a + (j - 1)* 2 = 5 + 2j

a + 2j - 2 = 5 + 2j

a - 2 = 5

a = 7

This means that the first term is 7, the second term is 9

Using the sum of nth terms of an arithmetic sequence, we have

S(n) = n/2 * (2a + (n - 1) * d)

So, we have

S(40) = 40/2 * (2 * 7 + (40 - 1) * 2)

S(40) = 1840

Subtracting the first and second terms, we have

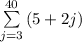`\sum\limits^(40)_(j=3) {(5 + 2j)}` = 1840 - 7 - 9

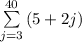`\sum\limits^(40)_(j=3) {(5 + 2j)}` = 1824

Hence, the value of the summation is 1824