Question

Please helpppppp, I don’t understand how to do this at alllll

Answer 1

`\textsf{3)}\quad y = -2x + 4`

`\textsf{4)}\quad y = (5)/(4)x - 4`

5) See attachment 1.

6) See attachment 2.

Explanation:

The slope-intercept form of a linear equation is:

`\boxed{\begin{array}{l}\underline{\textsf{Slope-intercept form of a linear equation}}\\\\\large\text{$y=mx+b$}\\\\\textsf{where:}\\\phantom{ww}\bullet\;\;\textsf{$m$ is the slope.}\\\phantom{ww}\bullet\;\;\textsf{$b$ is the $y$-intercept.}\\\end{array}}`

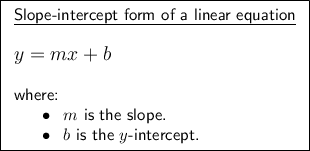
The slope (m) is the ratio of the vertical change (y) to the horizontal change (x) between two points on a graph or a curve. In other words, the slope measures how much y changes for a given change in x.

The y-intercept (b) is the y-value of the point where the graph intersects the y-axis.

`\hrulefill`

Graph 3

For each one-unit increase in x-values, the y-value decreases by 2 units, so the slope is m = -2. The line intersects the y-axis at y = 4, so b = 4.

Therefore, the equation for this line is:

`\large\boxed{\boxed{y = -2x + 4}}`

`\hrulefill`

Graph 4

For each 4-unit increase in x-values, the y-value increases by 5 units, so the slope is m = 5/4. The line intersects the y-axis at y = -4, so b = -4.

Therefore, the equation for this line is:

`\large\boxed{\boxed{y = (5)/(4)x - 4}}`

`\hrulefill`

Graph 5

Comparing the given equation y = 2x - 1 with the slope-intercept form, we find:

• Slope: m = 2
• y-intercept: b = -1

The y-intercept is the point at which the line intersects the y-axis, so its corresponding x-coordinate is always zero. Therefore, the y-intercept is (0, -1).

Given that the slope of the line is 2, this means that a one-unit increase in x-values corresponds to a 2-unit increase in y-values. To determine another point on the line, simply add 1 unit to the x-coordinate of the y-intercept, and add 2 units to the y-coordinate:

`(0 + 1 , -1 + 2) = (1, 1)`

Therefore, to graph the line of the equation y = 2x - 1, plot points (0, -1) and (1, 1) and draw a straight line through them. (See attachment 1).

`\hrulefill`

Graph 6

Comparing the given equation y = (3/5)x + 4 with the slope-intercept form, we find:

• Slope: m = 3/5
• y-intercept: b = 4

The y-intercept is the point at which the line intersects the y-axis, so its corresponding x-coordinate is always zero. Therefore, the y-intercept is (0, 4).

Given that the slope of the line is 3/5, this means that a 5-unit increase in x-values corresponds to a 3-unit increase in y-values. To determine another point on the line, simply add 5 units to the x-coordinate of the y-intercept, and add 3 units to the y-coordinate:

`(0 + 5 ,4+3) = (5,7)`

As this point is out of range of the given coordinate graph, we can find another point by subtracting 5 units from the x-coordinate of the y-intercept, and subtracting 3 units from the y-coordinate:

`(0 - 5 ,4-3) = (-5,1)`

Therefore, to graph the line of the equation y = (3/5)x + 4, plot points (0, 4) and (-5, 1) and draw a straight line through them. (See attachment 2).

Answer 2

See below

Explanation:

5)

To graph the lines
`\sf y = 2x - 1`, we can choose two points for each line and then connect them to form the lines.

For
`\sf y = 2x - 1`:

1. When
`\sf x = 0`,
`\sf y = 2(0) - 1 = -1`. So, one point is
`\sf (0, -1)`.

2. When
`\sf x = 1`,
`\sf y = 2(1) - 1 = 1`. So, another point is
`\sf (1, 1)`.

Plot the points and make a straight line passing through it.

For Graph: see Attachment

6)

To graph the lines
`\sf y = (3)/(5)x + 4`, we can choose two points for each line and then connect them to form the lines.

For
`\sf y = (3)/(5)x + 4`:

1. When
`\sf x = 0`,
`\sf y = (3)/(5)(0) + 4 = 4`. So, one point is
`\sf (0, 4)`.

2. When
`\sf x = 5`,
`\sf y = (3)/(5)(5) + 4 = 7`. So, another point is
`\sf (5, 7)`.

Plot the points and make a straight line passing through it.

For Graph: see Attachment