Answer:
-13vx

Explanation:
v
 - 6x
- 6x
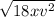
Rewrite 50x³ as (5x)²⋅ (2x)
v
 - 6x
- 6x
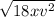
Pull terms out from under the radical.
v (5x
 ) - 6x
) - 6x
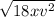
Rewrite using the commutative property of multiplication.
5vx
 - 6x
- 6x
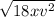
Rewrite 18xv² as (3v)² · (2x)
5vx(
 ) - 18vx (
) - 18vx (
 )
)
-13vx

So, the answer is -13vx
