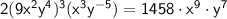Answer:
3.
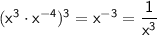
4.
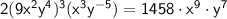
Explanation:
Let's simplify each expression step by step:
3.)

Apply the power rule for exponents, which states that
 :
:
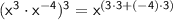
Simplify the exponent:
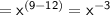
The expression
 can also be expressed as
can also be expressed as
 .
.
4.)
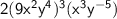
Apply the power rule again, distributing the exponents to both the base and the exponent outside the parentheses:
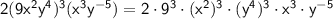
Simplify the coefficients and exponents:

Combine the x-terms by adding their exponents:
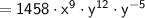
Combine the y-terms by subtracting their exponents:
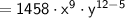
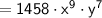
So, the simplified expressions are:
3.
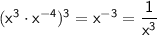
4.