Question

What’s the answer? I don’t understand it and an answer to the question would help me understand it more because there is a whole page of these

Answer 1

10)
`\sf x = -8`

11)
`\sf x = 4`

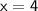
Explanation:

10)

Co-interior angles are angles that are located on the same side of a transversal and between two parallel lines. Co-interior angles are supplementary, which means that they add up to 180 degrees.

In the given case,
`\sf x + 78` and
`\sf x + 118` are co-interior angles. This means that their measures add up to 180 degrees.

Therefore, we can write the equation:

`\sf x + 78 + x + 118 = 180^\circ`

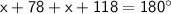
Combining like terms, we get:

`\sf 2x + 196 = 180^\circ`

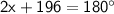
Subtracting 196 from both sides of the equation, we get:

`\sf 2x + 196-196 =( 180-196)^\circ`

`\sf 2x = -16`

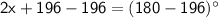
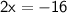
Dividing both sides of the equation by 2, we get:

`\sf (2x )/(2)=( -16)/(2)`

`\sf x = -8`

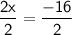
Therefore, the measures of x is -8.

11)

Alternate angles are angles that are located on the opposite sides of a transversal and between two parallel lines. Alternate angles are congruent, which means that they have the same measure.

In the given case,
`\sf 12x + 2` and
`\sf 50^\circ` are alternate angles.

This means that their measures are equal.

Therefore, we can write the equation:

`\sf 12x + 2 = 50^\circ`

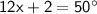
Subtracting 2 from both sides of the equation, we get:

`\sf 12x + 2-2 =( 50-2)^\circ`

`\sf 12x = 48`

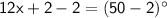
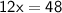
Dividing both sides of the equation by 12, we get:

`\sf ( 12x )/(12)= (48)/(12)`

`\sf x = 4`

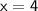
Therefore, the measure of x is 4.