Question

Use polynomial identities to factor 27x^3 – 64.

Answer 1

Answer:
`(3x-4)(9x^2+12x+16)`

Explanation:

The given expression,
`\(27x^3 - 64\)`, can be factored using the difference of cubes identity. The difference of cubes identity is given by:

`\[ a^3 - b^3 = (a - b)(a^2 + ab + b^2) \]`

In this case,
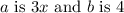`\(a\) is \(3x\) and \(b\) is \(4\)`. So, applying the difference of cubes formula, we have:

`\[ 27x^3 - 64 = (3x - 4)((3x)^2 + (3x)(4) + 4^2) \]`

Now, simplify the expression:

`\[ 27x^3 - 64 = (3x - 4)(9x^2 + 12x + 16) \]`

So, the factored form of
`\(27x^3 - 64\) is \((3x - 4)(9x^2 + 12x + 16)\).`