Question

Divide radicals + complex numbers

Answer 1

`\sf (3√(2) - 4)/(8)`

Explanation:

To divide the given expression involving radicals, we can rationalize the denominator. The expression is:

`\sf (3 - 2√(2))/(2√(8))`

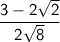
First, simplify the expression in the denominator:

`\sf 2√(8) = 2 * √(4 * 2) = 2 * 2√(2) = 4√(2)`

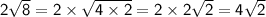
Now, rewrite the expression with the simplified denominator:

`\sf (3 - 2√(2))/(4√(2))`

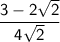
To rationalize the denominator, multiply both the numerator and denominator by the conjugate of the denominator.

The conjugate of
`\sf 4√(2)` is
`\sf 4√(2)`:

`\sf (3 - 2√(2))/(4√(2)) * (4√(2))/(4√(2))`

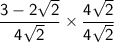
Now, multiply the numerators and denominators:

`\sf ((3 - 2√(2)) * 4√(2))/(4√(2) * 4√(2))`

Simplify:

`\sf (12√(2) - 8 * 2)/(16 * 2)`

`\sf (12√(2) - 16)/(32)`

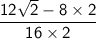
The final simplified expression is:

`\sf (12√(2) - 16)/(32)`

If we want to express this in its simplest form, we can factor out a common factor of 4 from the numerator:

`\sf (4(3√(2) - 4))/(32)`

Simplify further by canceling common factors:

`\sf (3√(2) - 4)/(8)`

So,
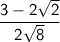`\sf (3 - 2√(2))/(2√(8))` simplifies to
`\sf (3√(2) - 4)/(8)`.

Answer 2

`\cfrac{3√(2) -4}{8}`

===================

Simplify in below steps:

• 
  `\cfrac{3-2√(2) }{2√(8) } =` Given
• 
  `\cfrac{3-2√(2) }{2*2√(2) } =` Simplify the denominator
• 
  `\cfrac{3-2√(2) }{4√(2) } =`
• 
  `\cfrac{√(2) (3-2√(2)) }{√(2) (4√(2) )} =` Multiply by
  `√(2)`
• 
  `\cfrac{3√(2) -4}{8}` Answer