Question

Please help i am super confused on what to do :)

Please explain how you got your answer too

Dont write something dum because i will report it i just need help on this question​

Answer 1

About 9.1 yards tall.

Explanation:

So, we can draw the right triangle shown below:

We want to find h.

Since we know the angle and the side adjacent to the angle and we wan to find the opposite side, we will use the tangent ratio:

`\displaystyle \tan(x^\circ)=\frac{\text{Opposite}}{\text{Adjacent}}`

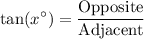
So:

`\displaystyle \tan(20)=(h)/(25)`

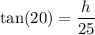
Therefore:

`\displaystyle h=25\tan(20)`

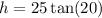
Use a calculator. Ensure your calculator is in the correct mode (degrees mode):

`h\approx 9.1\text{ yards}`

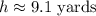
The goal post is around 9.1 yards tall.