Question

Y=-2x + 4 3y + 6x = 12 O One Solution O No Solutions o Infinitely Many Solutions

Answer 1

To find the number of solutions of a system of linear equations you need to identify the slope (m) of each equation

`y=mx+b`

If the slope is the same in both lines the system has no solution.

If the slope is different in the lines the system has one solution.

If the equation are the same (incluided the value of b) the system has infinitely many solutions.

In the given equations the slope is:

First equation:

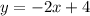
`y=-2x+4`Slope: m=-2

Second equation:

`3y+6x=12`

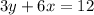
Write the equation in slope-intercept form by solving for y:

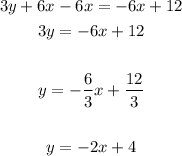
`\begin{gathered} 3y+6x-6x=-6x+12 \\ 3y=-6x+12 \\ \\ y=-(6)/(3)x+(12)/(3) \\ \\ y=-2x+4 \end{gathered}`Slope: m= -2In this case as the equatinos are the same the system has infinitely many solutions