Given that 'M' varies directly with the square of 'd',
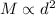
Given that 'M' varies inversely with the square root of 'x',
![M\propto\frac{1}{\sqrt[]{x}}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/ruftf9zew1mwp5m4jayz.png)
Combining the relationships,
![M\propto\frac{d^2}{\sqrt[]{x}}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/i566mjtpz3yf1eeoogsq.png)
Let 'k' be the constant of proportionality. Then,
![M=k\cdot\frac{d^2}{\sqrt[]{x}}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/mtc3y61nfb1yrtnks6zt.png)
Given that M=12 when d=3 and x=4,
![\begin{gathered} 12=k\cdot\frac{(3)^2}{\sqrt[]{4}} \\ 12=k\cdot(9)/(2) \\ k=(12\cdot2)/(9) \\ k=(8)/(3) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/85s4k5p9hwvpff33ex8y.png)
Substitute the value of this constant in the general expression,
![M=(8)/(3)\cdot\frac{d^2}{\sqrt[]{x}}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/822xpo8lh8jtlu6apj50.png)
Thus, the required general formula to describe the relation is obtained as,
![M=(8)/(3)\cdot\frac{d^2}{\sqrt[]{x}}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/822xpo8lh8jtlu6apj50.png)