Question

Please help me with this problem:The data shows the number of grams of fat found in 9 different health bars.11, 11.5, 10.5, 17, 14.5, 14.5, 18, 17, 19What is the IQR (interquartile range) for the data? 6.25714.517.5

Answer 1

Answer:

The interquartile range = 6.25

Step-by-step explanation:

The given dataset is:

11, 11.5, 10.5, 17, 14.5, 14.5, 18, 17, 19

Rearrange the data in ascending order

10.5, 11, 11.5, 14.5, 14.5, 17, 17, 18, 19

The number of terms in the data, N = 9

The lower quartile is calculated as:

`\begin{gathered} Q_1=((N+1)/(4))^(th)term \\ \\ Q_1=(9+1)/(4)^(th)term \\ \\ Q_1=2.5th\text{ term} \\ \\ Q_1=(11+11.5)/(2) \\ \\ Q_1=(22.5)/(2) \\ \\ Q_1=11.25 \end{gathered}`

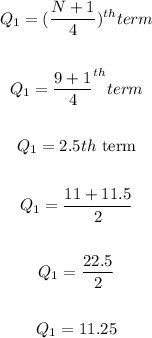
The upper quartile is calculated as:

`\begin{gathered} Q_3=((3(N+1))/(4))^{th\text{ }}term \\ \\ Q_3=(3(9+1))/(4)th\text{ terms} \\ \\ Q_3=7.5th\text{ term} \\ \\ Q_3=(17+18)/(2) \\ \\ Q_3=17.5 \end{gathered}`

The interquartile range = Upper quartile - Lower quartile

The interquartile range = 17.5 - 11.25

The interquartile range = 6.25