Question

A quadratic function and an exponential function are graphed below. How do the decay rates of the functions compareover the interval -2

Answer 1

To check the decay rate, we need to check the variation in y-axis.

Since our interval is

To compare the decay rates we need to check the variation on the y-axis of both functions.

`\begin{gathered} \Delta y_1=f(-2)-f(0)=4-1=3 \\ \Delta y_2=g(-2)-g(0)=4-0=4 \end{gathered}`

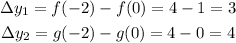
Now, we calculate their ratio to find how they compare:

`(\Delta y_1)/(\Delta y_2)=(3)/(4)`

This tell us that the exponential function decays at three-fourths the rate of the quadratic function.

And this is the fourth option.