Question

Find the equation of the line that has slope -2/3 and which passes through ​(-1​,-5​).

Answer 1

y = ⅔x - 5⅔

Explanation:

y=mx+b, where m is the slope, and b is the y-intercept.

The slope is given, and the point (-1,-5) is known. From that information, we can continue the line to find the y-intercept. The second point can be plotted at (2,-7), making the y-intercept -5⅔.

We can now substitute the variables in the equation to get:

y = ⅔x - 5⅔

Answer 2

`y+5=-(2)/(3) (x+1)`

Explanation:

Pre-Solving

We are given that a line passes through (-1, -5) and has a slope (m) of
`-(2)/(3)`.

We want to write the equation of it.

There are 3 ways to write the equation of the line:

• Slope-intercept form, which is y=mx+b where m is the slope and b is the y-intercept.
• Standard form, which is ax+by=c, where a, b, and c are free integer coefficients but a and b cannot be 0.

1. Point-slope form, which is
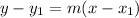   `y-y_1=m(x-x_1)` where m is the slope and
   `(x_1,y_1)` is a point.

As the question doesn't specify, we can use any one of the forms. However, let's write the equation in point-slope form, as that would be easiest for us.

Solving

First, substitute
`-(2)/(3)` as m in the equation.

`y-y_1=-(2)/(3) (x-x_1)`

Now, substitute
`-1` as
`x_1` and -5 as
`y_1`.

`y--5=-(2)/(3) (x--1)`

`y+5=-(2)/(3) (x+1)`