Question

The electrical resistance of a wire varies directly with the length of the wire and inversely with the square of the diameter of the wire. If a wire 432 feet long and 4 millimeters in diameter has a resistance of 1.26 ohms, find the length of a wire of the same material whose resistance is ohms and whose diameter is millimeters.

Answer 1

Let

R ----> resistance in ohms

L ---> the length of the wire in ft

D ---> the diameter of the wire in mm

In this problem, the equation is of the form

`R=K(L)/(D^2)`

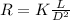
we have

L=432 ft

D=4 mm

R=1.26 ohms

so

Find out the value of K (constant of proportionality)

substitute the given values

`\begin{gathered} 1.26=K(432)/(4^2) \\ \\ K=(1.26*16)/(432) \\ \\ K=0.0467 \end{gathered}`

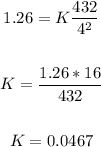
Part 2

The formula is

`R=0.0467(L)/(D^2)`

For

R=1.41 ohms

D=5 mm

substitute in the formula above

`\begin{gathered} 1.41=0.0467(L)/(5^2) \\ solve\text{ for L} \\ L=(1.41*25)/(0.0467) \\ L=754.8\text{ ft} \end{gathered}`

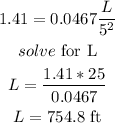
The answer is 754.8 feet