Answer:
a) 0.7696 = 76.96% probability that at least 11 of them graduated.
b) 0.0474 = 4.74% probability that exactly 9 of them graduated.
c) Since 9 is less than 2.5 standard deviations from the mean, it would not be unusual.
Explanation:
For each student, there are only two possible outcomes. Either they graduated, or they did not. Each student is independent of each other. So we use the binomial probability distribution to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.
The expected value of the binomial distribution is:
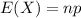
The standard deviation of the binomial distribution is:
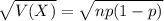
Unusual outcome:
An outcome in the binomial distribution is unusual if it is more than 2.5 standard deviations from the mean.
92.4% for the medical students admitted through special programs.
This means that
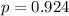
a. If 12 of the students from the special programs are randomly selected, find the probability that at least 11 of them graduated.
This is
 when
when
 . So
. So
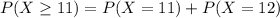
In which

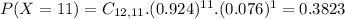
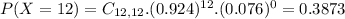
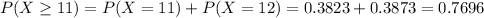
0.7696 = 76.96% probability that at least 11 of them graduated.
b. If 12 of the students from the special programs are randomly selected, find the probability that exactly 9 of them graduated.
This is P(X = 9). So


0.0474 = 4.74% probability that exactly 9 of them graduated.
c. Would it be unusual to randomly select 12 students from the special programs and get exactly 9 that graduate?
The mean number of students expected to graduate is:
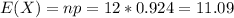
The standard deviation is
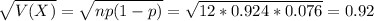
11.09 - 2.5*0.92 = 8.79
This means that anything below 8.79 is unusual, so, since 9 is less than 2.5 standard deviations from the mean, it would not be unusual.