Question

Hi I don’t understand this question,can u do it step by step? Thanks!

Answer 1

The rule of the division of differentiation is

`(d)/(dx)((u)/(v))=(u^(\prime)v-uv^(\prime))/(v^2)`

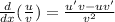
The given function is

`y=f(x)=(x^2+3x+3)/(x+2)`

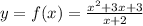
a)

Let u the numerator and v the denominator

`\begin{gathered} u=x^2+3x+3 \\ u^(\prime)=2x+3 \end{gathered}`
`\begin{gathered} v=x+2 \\ v^(\prime)=1 \end{gathered}`

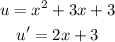
Substitute them in the rule above

`\begin{gathered} (dy)/(dx)=((2x+3)(x+2)-(x^2+3x+3)(1))/((x+2)^2) \\ (dy)/(dx)=(2x^2+7x+6-x^2-3x-3)/((x+2)^2) \\ (dy)/(dx)=(x^2+4x+3)/((x+2)^2) \\ (dy)/(dx)=((x+3)(x+1))/((x+2)^2) \end{gathered}`

We will differentiate dy/dx again to find d^2y/dx^2

`\begin{gathered} u=x^2+4x+3 \\ u^(\prime)=2x+4 \end{gathered}`
`\begin{gathered} v=(x+2)^2=x^2+4x+4 \\ v^(\prime)=2x+4 \end{gathered}`

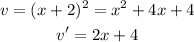
Then substitute them in the rule above

`\begin{gathered} (d^2y)/(dx^2)=((2x+4)(x^2+4x+4)-(x^2+4x+3)(2x+4))/((x^2+4x+4)^2) \\ (d^2y)/(dx^2)=((2x+4)\lbrack x^2+4x+4-x^2-4x-3\rbrack)/((x^2+4x+4)^2) \\ (d^2y)/(dx^2)=((2x+4)\lbrack1\rbrack)/((x^2+4x+4)^2) \\ (d^2y)/(dx^2)=((2x+4))/((x+2)^4) \\ (d^2y)/(dx^2)=(2(x+2))/((x+2)^4) \\ (d^2y)/(dx^2)=(2)/((x+2)^3) \end{gathered}`

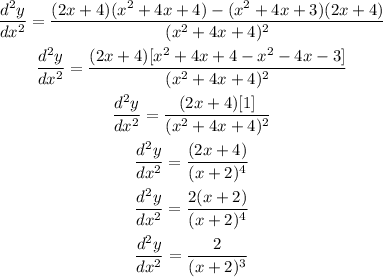
b)

The turning point is the point that has dy/dx = 0

Equate dy/dx by 0 to find the values of x

`\begin{gathered} (dy)/(dx)=((x+3)(x+1))/((x+2)^2) \\ (dy)/(dx)=0 \\ ((x+3)(x+1))/((x+2)^2)=0 \end{gathered}`

By using the cross multiplication

`\begin{gathered} (x+3)(x+1)=0 \\ x+3=0,x+1=0 \\ x+3-3=0-3,x+1-1=0-1 \\ x=-3,x=-1 \end{gathered}`

Substitute x by -3 and -1 in f(x) to find y

`\begin{gathered} f(-3)=((-3)^2+3(-3)+3)/(-3+2) \\ f(-3)=(3)/(-1) \\ y=-3 \end{gathered}`
`\begin{gathered} f(-1)=((-1)^2+3(-1)+3)/(-1+2) \\ f(-1)=(1)/(1) \\ y=1 \end{gathered}`

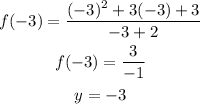
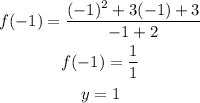
The turning points are (-3, -3) and (-1, 1)

c)

To find the inflection point equate d^2y/dx^2 by 0 to find x

`\begin{gathered} (d^2y)/(dx^2)=(2)/((x+2)^3) \\ (d^2y)/(dx^2)=0 \\ (2)/((x+2)^3)=0 \end{gathered}`

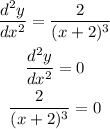
By using the cross multiplication

`2=0`

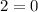
Which is wrong 2 can not be equal to zero, then

NO inflection point for the curve

d)

Since the denominator of the curve is x + 2, then

Equate it by 0 to find the vertical asymptote

`\begin{gathered} x+2=0 \\ x+2-2=0-2 \\ x=-2 \end{gathered}`

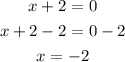
There is a vertical asymptote at x = -1

Since the greatest power of x up is 2 and the greatest power of down is 1, then there is an Oblique asymptote by dividing up and down

`\begin{gathered} (x^2+3x+3)/(x+2)=x+1 \\ y=x+1 \end{gathered}`

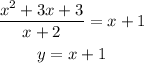
The Oblique asymptote is y = x + 1

No horizontal asymptote

e)

This is the graph of y = f(x)

This is the graph of y = f(IxI)

f)

For the curve

`y=(x^2-3x+3)/(2-x)`

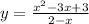
Take (-) sign as a common factor down, then

`\begin{gathered} y=((x^2+3x+3))/(-(-2+x)) \\ y=-((x^2-3x+3))/((x-2)) \end{gathered}`

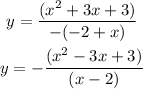
Since the sign of y is changed, then

`y=-f(x)`

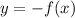
Then it is the reflection of f(x) about the y-axis we can see it from the attached graph

The red graph is f(x)

The purple graph is -f(x) which is the equation of the last part