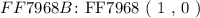Question

Point M is the midpoint of AB. The coordinates of point A are (-7,2) and the coordinates of M are (-3, 1).What are the coordinates of point B?The coordinates of point B are

Answer 1

We have three points that lie on a line namely, A M B, in a cartesian coordinate system.

The coordinates of each point are as such:

`A\text{ ( -7 , 2 )}`

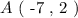
The mid-point of a line segment ( AB ) is given by coordinate ( M ) as follows:

`M\text{ ( -3 , 1 )}`

We will define the coordinates of point ( B ) in terms of cartesian coordinates as follows:

`B\text{ ( x , y ) }`

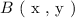
Here the line ( A B ) with points [ A, M , B ] are colinear. This means they all lie on the same line passing through all three points.

A straight line always have a constant slope/gradient ( m ) . This slope is determined between two points that lie on the line.

The formulation of calculating the slope ( m ) of the line in a cartesian coordinate system is as follows:

`m\text{ = }(y_2-y_1)/(x_2-x_1)`

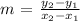
The respective cartesian coordinates of each of the two points are represented by sub-scripts of ( x and y ).

Here we will take two points ( A and M ) to determine the slope ( m ) of the line passing throgh all three points as follows:

`\begin{gathered} m\text{ = }\frac{1\text{ - 2}}{-3\text{ -(-7)}}\text{ = }(1-2)/(-3+7) \\ m\text{ = }(-1)/(4) \end{gathered}`

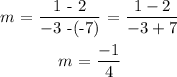
This slope ( m ) holds true for the entire line and must satisfy all consecutive points that lie on the line.

We will now consider points ( A and B ) and determine the slope ( m ):

`\begin{gathered} m\text{ = }\frac{y\text{ - 2}}{x\text{ - (-7)}} \\ \\ -(1)/(4)\text{ = }\frac{y\text{ - 2}}{x+7} \\ \\ -\text{ ( x + 7 ) = 4}\cdot(y-2) \\ -x\text{ - 7 = 4y - 8} \\ \textcolor{#FF7968}{x}\text{\textcolor{#FF7968}{ = 1 - 4y }}\textcolor{#FF7968}{\ldots}\text{\textcolor{#FF7968}{ Eq 1}} \end{gathered}`

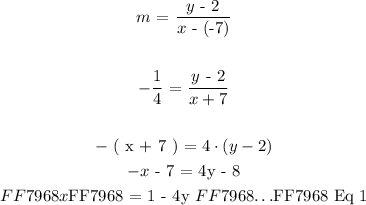
What we did above was calculated the slope ( m ) between two points ( A and B ) which resulted in an expression in terms of coordinates of point B ( x and y ). We equated that expression with the value of slope ( m ) that holds true for all points that lie on the line.

Then we used the slope equation and expressed the x-coordinate of point B in terms of y-coordinate of point B. This relaitonship is termed as Equation 1 ( Eq 1 ).

Next, we were also given that point M is the midpoint of line segment ( AB ). Using the definition of a mid-point ( M ) i.e the magnitude of line segments are:

`|AM|\textMB`

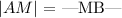
The magnitudes of line segment AM and MB must be equal for point ( M ) to be a mid-point of line segment ( AB ).

We will express the formulation of determining a magnitude of line segment using two points:

`\text{length of line segment = }√((*_2-x_1)^2+(y_2-y_1)2)`

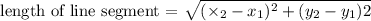
So for equating the lengths (magnitudes) of line segments ( AM ) and ( MB ) we have:

`\begin{gathered} A\text{ ( -7 , 2 ) , M ( -3 , 1 ) , B ( x , y )} \\ √((-3-(-7))^2+(1-2)^2)\text{ = }√((-3-x)^2+(1-y)^2) \end{gathered}`

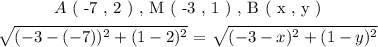
Square both sides of the equation:

`(4)^2+(-1)^2=(-1)^2\cdot(x+3)^2+(1-y)^2`

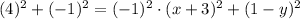
Evaluate left hand side of equation and apply PEMDAS at right hnd side of the equation:

`\begin{gathered} 16+1=(x^2+6x+9)+(y^2\text{ - 2y + 1 )} \\ 17\text{ = }x^2+y^2\text{ + 6x - 2y + 10} \\ \textcolor{#FF7968}{7}\text{\textcolor{#FF7968}{ = }}\textcolor{#FF7968}{x^2+y^2}\text{\textcolor{#FF7968}{ + 6x - 2y }}\textcolor{#FF7968}{\ldots Eq2} \end{gathered}`

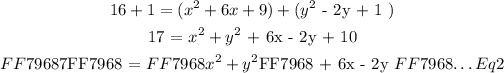
We have two equations with two unknows ( x and y ) as follows:

`\begin{gathered} x\text{ = 1 - 4y }\ldots Eq1 \\ 7=x^2+y^2\text{ + 6x - 2y }\ldots Eq2 \end{gathered}`

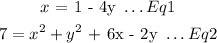
We will solve the two equation by simultaneous substitution method. Substitute Eq1 into Eq2 as follows:

`\begin{gathered} 7=(1-4y)^2+y^2\text{ + 6}\cdot(1\text{ - 4y ) - 2y} \\ 7=(1-8y+16y^2)+y^2\text{ + ( 6 - 24y ) - 2y} \\ 7=17y^2\text{ -34y + 7 } \\ 0=17y^2\text{ -34y} \\ 0\text{ = y}\cdot(17y\text{ - 34 )} \\ \textcolor{#FF7968}{y}\text{\textcolor{#FF7968}{ = 0 OR y = }}\textcolor{#FF7968}{(34)/(17)}\text{\textcolor{#FF7968}{ = 2}} \end{gathered}`

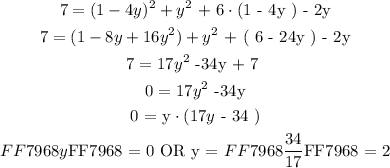
We will now plug the two values of coordinate ( y ) into ( Eq 1 ) and solve for ( x ):

`\begin{gathered} y\text{ = 0, x = 1 - 4}\cdot(0)\text{ = 1} \\ y\text{ = 2, x = 1 -4}\cdot(2)=-7\text{ } \end{gathered}`

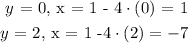
We have two solutions for the coordinates of point ( B ) as follows:

`B\text{ : ( 1 , 0 ) OR ( -7 , 2 )}`

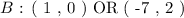
However, point B must have only one pair of coordinate. So we have to investigate both solutions given above and reject a redundant solution.

We see that solution B: ( -7 , 2 ) is redundant solution, hence, rejected. This is because it represents the coordinates of point A: ( -7 , 2 ) - given in question. So two different points can not attain the same set of coordinates! Hence,

The solution to the set of coordinates of point B is:

`\textcolor{#FF7968}{B\colon}\text{\textcolor{#FF7968}{ ( 1 , 0 ) }}`