Question

Ms. Burns is at a window in a building and spots her worst enemy in a building across an 7.1m wide alley. If she throws a water balloon directly horizontally at a velocity of 6.35m/s, how far below her can her enemy be and still hit them?

Answer 1

6.132 meters

Step-by-step explanation:

To solve this problem, we can use the following steps:

1. Identify the known and unknown quantities.

Known quantities:

• Horizontal velocity of the water balloon, u = 6.35 m/s
• Width of the alley, w = 7.1 m

Unknown quantity:

• Maximum distance the water balloon can fall, h

2. Choose the appropriate equation of motion.

Since the water balloon is moving horizontally in free fall, we can use the following equation of motion:

`\boxed{\boxed{\sf h = (1)/(2)\cdot g \cdot t^2} }`

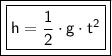
where:

• h is the distance fallen
• g is the acceleration due to gravity (9.81 m/s^2)
• t is the time taken to fall

3. Solve for the unknown quantity.

We can rearrange the equation to solve for h:

`\sf h = (1)/(2)\cdot g \cdot t^2`

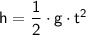
To find t, we can use the following equation of motion for horizontal motion:

`\sf w = ut`

where:

• w is the distance traveled
• u is the initial velocity
• t is the time taken to travel the distance

Substituting in the known values, we get:

`\sf 7.1 m = 6.35 m/s \cdot t`

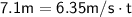
Solving for t, we get:

`\sf t =(7.1)/(6.35)`

`\sf t = 1.1181102362204 s`

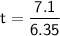
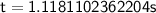
Substituting this value back into the equation for h, we get:

`\sf h = (1)/(2)\cdot 9.81 \cdot (1.1181102362204)^2`

`\sf h = 6.1320863041718137650195300648 m`

`\sf h = 6.132\textsf{ m in 3 d.p)}`

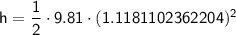
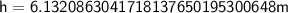
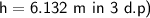
Therefore, Ms. Burns' enemy can be up to 6.132 meters below her and she will still hit them with the water balloon.