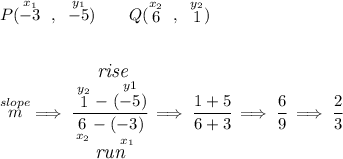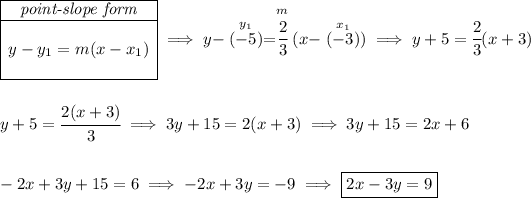Question

A line passes through the points $P$ and $Q.$ If $P = (-3,-5)$ and $Q = (6,1),$ then write the equation of this line in the form $Ax + By = C,$ where $A$, $B$, and $C$ are integers with greatest common divisor $1,$ and $A$ is positive.

Answer 1

standard form for a linear equation means

• all coefficients must be integers, no fractions

• only the constant on the right-hand-side

• all variables on the left-hand-side, sorted

• "x" must not have a negative coefficient

to get the equation of any straight line, we simply need two points off of it, let's use those two provided.

`P(\stackrel{x_1}{-3}~,~\stackrel{y_1}{-5})\qquad Q(\stackrel{x_2}{6}~,~\stackrel{y_2}{1}) \\\\\\ \stackrel{slope}{m}\implies \cfrac{\stackrel{\textit{\large rise}} {\stackrel{y_2}{1}-\stackrel{y1}{(-5)}}}{\underset{\textit{\large run}} {\underset{x_2}{6}-\underset{x_1}{(-3)}}} \implies \cfrac{1 +5}{6 +3} \implies \cfrac{ 6 }{ 9 } \implies \cfrac{2}{3}`

`\begin{array}ll \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{(-5)}=\stackrel{m}{\cfrac{2}{3}}(x-\stackrel{x_1}{(-3)}) \implies y +5 = \cfrac{2}{3} ( x +3) \\\\\\ y +5 = \cfrac{2( x +3)}{3} \implies 3y+15=2(x+3)\implies 3y+15=2x+6 \\\\\\ -2x+3y+15=6\implies -2x+3y=-9\implies \boxed{2x-3y=9}`