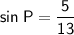Question

TRIG HELP!!

Find the value of sin P rounded to the nearest hundredth, if necessary.

Answer 1

0.38 (rounded)

Explanation:

We can use the Pythagorean theorem to find the hypotenuse.

`5^2+12^2 = c^2\\25 +144 = c^2\\169 = c^2\\13 = c`

We can now find sin(p). Recall sin is the "opposite over hypotenuse."

`sin((5)/(13)) = 0.375`

Answer 2

`\sf sin\: P = (5 )/( 13)`

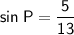
Explanation:

Given:

In ∆ PQR

• PQ = 12
• QR = 5

To find:

• sin P

Solution:

To find sin P, we can use the following formula:

`\boxed{\boxed{\sf sin \: P =(Opposite )/(Hypotenuse) = (QR )/( PR)}}`

We know that QR = 5 and that PR = the hypotenuse of the triangle. We can use the Pythagorean Theorem to find PR:

`\begin{aligned} PR^2 & = PQ^2 + QR^2 \\\\ PR^2 & = 12^2 + 5^2 \\\\ PR^2 & = 169 \\\\ PR & =√(169)\\\\ PR & = 13 \end{aligned}`

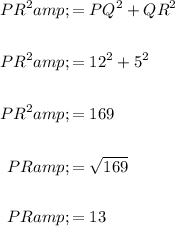
Now that we know the values of QR and PR, we can plug them into the formula for sin P:

`\sf sin\: P =( QR )/(PR) = (5 )/( 13)`

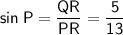
Therefore,
`\sf sin\: P = (5 )/( 13)`