The correct answer is option C)
 .
.
To find the ratio of magnetic induction
 when the number of turns in a circular coil changes from 2 to 5 while the current remains the same, we can use the formula for the magnetic field inside a circular coil:
when the number of turns in a circular coil changes from 2 to 5 while the current remains the same, we can use the formula for the magnetic field inside a circular coil:
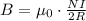
Where:
 = Magnetic induction
= Magnetic induction
 = Permeability of free space (a constant)
= Permeability of free space (a constant)
 = Number of turns in the coil
= Number of turns in the coil
 = Current
= Current
 = Radius of the circular coil
= Radius of the circular coil
Let's denote the initial coil as Coil 1 and the final coil as Coil 2.
For Coil 1:
 turns
turns
For Coil 2:
 turns (as it's rewound into a circular coil with 5 turns)
turns (as it's rewound into a circular coil with 5 turns)
The current
 is the same in both cases.
is the same in both cases.
Now, let's find the ratio of
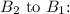
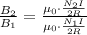
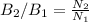
Substitute the values:
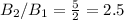
So, the correct answer is:
C)
