Answer:
m∠B =
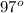 .
.
Explanation:
Since the three sides of the triangle are given, then we apply cosine rule.
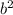 =
=
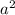 +
+
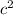 - 2ac Cos B
- 2ac Cos B
But, a = 660 cm, b = 680 cm, and c = 100 cm.
So that;
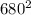 =
=
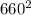 +
+
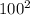 -2(660 x 100) Cos B
-2(660 x 100) Cos B
462400 = 435600 + 10000 - 132000 Cos B
462400 = 445600 - 132000 Cos B
132000 Cos B = 445600 - 462400
= -16800
Cos B =
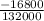
= -0.1273
B =
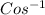 -0.1273
-0.1273
=
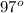
Thus, measure of ∠B is
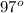 .
.