Question

Leo drew a line that is perpendicular to the line shown on the grid and passes through point (F, G). Which of the following is the equation of Leo's line?

Choices:
A. y − F = −2(x − G)y
B. y + F = 2(x + G)
C. y − G = −one half(x − F)
D. y − G = −one half(x + F)

Answer 1

keeping in mind that perpendicular lines have negative reciprocal slopes, let's check for the slope of the line in the grid above. Now to get the slope of any straight line, we simply need two points off of it, let's use those two in the picture below.

`(\stackrel{x_1}{-3}~,~\stackrel{y_1}{-4})\qquad (\stackrel{x_2}{1}~,~\stackrel{y_2}{4}) \\\\\\ \stackrel{slope}{m}\implies \cfrac{\stackrel{\textit{\large rise}} {\stackrel{y_2}{4}-\stackrel{y1}{(-4)}}}{\underset{\textit{\large run}} {\underset{x_2}{1}-\underset{x_1}{(-3)}}} \implies \cfrac{4 +4}{1 +3} \implies \cfrac{ 8 }{ 4 } \implies 2 \\\\[-0.35em] ~\dotfill`

`\stackrel{~\hspace{5em}\textit{perpendicular lines have \underline{negative reciprocal} slopes}~\hspace{5em}} {\stackrel{slope}{ 2 \implies \cfrac{2}{1}} ~\hfill \stackrel{reciprocal}{\cfrac{1}{2}} ~\hfill \stackrel{negative~reciprocal}{-\cfrac{1}{2} }}`

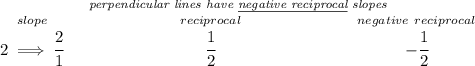
so we are really looking for the equation of a line whose slope is -1/2 and it passes through (F , G)

`(\stackrel{x_1}{F}~,~\stackrel{y_1}{G})\hspace{10em} \stackrel{slope}{m} ~=~ -\cfrac{1}{2} \\\\\\ \begin{array}ll \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{G}=\stackrel{m}{-\cfrac{1}{2}}(x-\stackrel{x_1}{F})`