Answer:
Approximately
 degrees (approximately
degrees (approximately
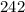 radians.)
radians.)
Step-by-step explanation:
The rotation of the wheels can be found in the following steps:
- Apply the SUVAT equations of linear motion to find the linear distance travelled.
- Divide the linear distance travelled by radius
 to find the angle of rotation.
to find the angle of rotation.
Assuming that the vehicle started from rest, such that initial velocity would be
 . At a constant linear acceleration of
. At a constant linear acceleration of
 , the linear displacement in
, the linear displacement in
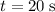 would be:
would be:
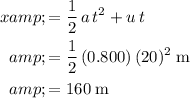 .
.
Since the direction of motion of this vehicle stays the same, the distance travelled would be equal to the magnitude of linear displacement:
 .
.
Divide the linear displacement by the radius to find the angle of rotation, in radians:
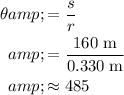 .
.
In other words, the angle of rotation would be approximately
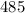 radians for each wheel.
radians for each wheel.
Radians and degrees are both units of measurement of angles. The relation between the two is
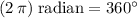 . Multiply the angle of rotation in radians by
. Multiply the angle of rotation in radians by
 to find the measurement in degrees:
to find the measurement in degrees:
 .
.
In other words, the angle of rotation is approximately.
 degrees.
degrees.