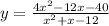Question

Can u help me fix what i did wrong in the equation

Answer 1

Based on the question, the vertical asymptote is x = -4 and x = 3. This means the denominator cannot have these x-values or else, the function becomes undefined. Hence, from these x-values, we can say that the factors of the denominator are:

`(x+4)(x-3)`

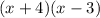
Multiplying the factors, we get:

`\begin{gathered} \Rightarrow x^2-3x+4x-12 \\ \Rightarrow x^2+x-12 \end{gathered}`

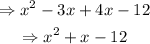
So, the denominator of our rational function must be x² + x - 12 in order to have those vertical asymptotes.

Another given information is that our x-intercepts are at x = -2 and x = 5. This means that the numerator must be zero at these x-values. Hence, we can say that some factors of the numerator are:

`(x+2)(x-5)`

Multiplying these two factors, we get:

`\begin{gathered} \Rightarrow x^2-5x+2x-10 \\ \Rightarrow x^2-3x-10 \end{gathered}`

This means x² - 3x - 10 should be part of our numerator.

Another given information is that the horizontal asymptote is at y = 4. This means that the ratio between the leading coefficients of the numerator and denominator is 4. (since both have the same degree)

So, in order to have a ratio of 4, we will multiply our numerator by 4.

`4(x^2-3x-10)\Rightarrow4x^2-12x-40`

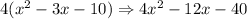
Therefore, our numerator must be 4x² - 12x - 40. And as mentioned above, the denominator must be x² + x - 12. So, the rational function is:

`y=(4x^2-12x-40)/(x^2+x-12)`