Question

find the inverse of each function. give any restrictions of the domain
`g(x) = - (2)/(* + 2) - 3`

Answer 1

The inverse function is

`g^(-1)(x)=(5x)/(4)+(25)/(4)`

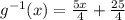
The domain of this inverse function is all real numbers.

Step-by-step explanation

The question asks us to find the invers of the given function and give any restrictions of the domain if that exists.

The function is

g(x) = -5 + (4x/5)

To obtain the inverse of a function, the right approach is to write g(x) as y, then make x the subject of formula.

`\begin{gathered} y=-5+(4x)/(5) \\ \text{Multiply through by 5} \\ 5y=-25+4x \\ \text{Rewrite the equation} \\ -25+4x=5y \\ 4x=5y+25 \\ \text{Divide through by 4} \\ (4x)/(4)=(5y)/(4)+(25)/(4) \\ x=(5y+25)/(4) \end{gathered}`

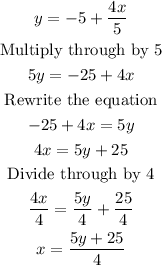
We can then write this properly in terms of the inverse function

`g^(-1)(x)=(5x)/(4)+(25)/(4)`

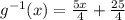
The domain of a function refers to the values of the independent variable (x), where the dependent variable [y or f(x)] or the function has a corresponding real value. The domain is simply the values of x for which the output also exists.

The domain of this inverse function is all real numbers because there would be a real number answer for every real number value of x.

Hope this Helps!!!