Question

College Algebra please help thank youu

Answer 1

Answer: 6

Explanation:

The question requires that you factor the expression
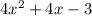`4x^2+4x-3` into the format
`(ax + b)(cx + d)` to extract the variables
`a, b, c, d` and add them together.

To factor the expression, we will use grouping where the 2nd term (
`bx` in
`ax^2+bx+c`, 4x in this case) is split into two terms so the equation can be "grouped" up by common factors.

`4x^2+4x-3`

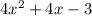
2 terms that multiply to a*c and add to b are 6 and -2 so we split the second term into the respective coefficients to get 6x and -2x.

`4x^2+6x-2x-3`

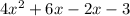
Now we find the common factors of each two terms we see. In this case, it is -1 and 2x.

`2x(2x+3)-(2x+3)`

Since we have a common factor of
`2x+3` and a factor of
`2x - 1`, we can create the following factored expression:

`(2x-1)(2x+3)`

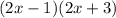
Using the
`(ax+b)(cx+d)` format provided, we can retrieve the variables from our new factored expression.

From our expression,
`a=2, b=-1, c=2, d=3`

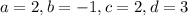
Now we add these terms together:

`a+b+c+d=2+(-1)+2+3=2-1+2+3=7-1=6`