Answer:
JT = 26
Explanation:
We are given the following information:
JT = 3x + 5
CT = 76
CJ = 6x + 8
We want to find JT so that CT = CJ + JT.
Since CT is a line where J lies in it. So,
CT = CJ + JT
Substitute the values:
76 = (6x + 8) + (3x + 5)
Now, combine like terms:
76 = 6x + 8 + 3x + 5
Combine the constants:
76 = (6x + 3x) + (8 + 5)
Combine like terms on both sides of the equation:
76 = 9x + 13
Now, isolate 9x by subtracting 13 from both sides of the equation:
76 - 13 = 9x
63 = 9x
Now, divide both sides by 9 to solve for x:
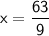
x = 7
Now that we've found the value of x, we can find JT:
JT = 3x + 5
JT = 3(7) + 5
JT = 21 + 5
JT = 26
So, JT = 26.