Question

A yearbook company promises to give the junior class a picnic if they spend at least $28,000 on yearbooks and class rings. Each yearbook, y, costs $35, and each class ring, r, costs $140. Write an inequality that represents this situation.

Answer 1

`\sf \boxed{\sf 35y + 140r \geq 28,000 }`

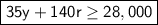
Explanation:

Let the total cost for selling yearbooks = 35y and total cost for selling class rings = 140r

And total cost = 35y + 140r

According to the question, the company will give picnic to the junior class if they spend at least $28000.

So, we can create the following inequality:

`\sf \boxed{\sf 35y + 140r \geq 28,000 }`

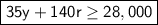
In this inequality:

• 35y represents the cost of the yearbooks (each yearbook costs $35, and there are y yearbooks).
• 140r represents the cost of the class rings (each class ring costs $140, and there are r class rings).
• 28,000 represents the minimum amount the junior class needs to spend.

The inequality states that the total cost of yearbooks and class rings must be greater than or equal to $28,000 for the junior class to receive the promised picnic.