Question

Graph the function and make a X and Y table of all points.

Answer 1

See the attached graph.

Step-by-step explanation:

To graph the function y = √(x + 6) - 3 and create an x and y table of points within the specified range, choose a range of x-values, calculate the corresponding y-values, and then plot the points on a graph.

Since the value under the square root sign cannot be negative, the domain of the function is restricted to x ≥ -6. Therefore, the range of x-values for the given coordinate plane is -6 ≤ x ≤ 8. Calculate the corresponding y-values (rounded to one decimal place) by substituting the x-values into the given function:

`\begin{array}\cline{1-2}x&y\\\cline{1-2}-6&-3\\\cline{1-2}-5&-2\\\cline{1-2}-4&-1.6\\\cline{1-2}-3&-1.3\\\cline{1-2}-2&-1\\\cline{1-2}-1&-0.8\\\cline{1-2}0&-0.6\\\cline{1-2}1&-0.4\\\cline{1-2}2&-0.2\\\cline{1-2}3&0\\\cline{1-2}4&0.2\\\cline{1-2}5&0.3\\\cline{1-2}6&0.5\\\cline{1-2}7&0.6\\\cline{1-2}8&0.7\\\cline{1-2}\end{array}`

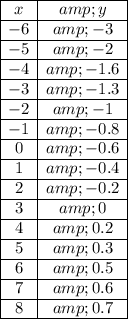
Plot the following points on the given coordinate plane:

• (-6, -3)
• (-5, -2)
• (-2, -1)
• (3, 0)
• (8, 0.7)

Draw a smooth curve from (-6, -3) through the plotted points. Add an arrow to the end of the curve at (8, 0.7) to indicate that the curve continues in that direction.

The function starts in quadrant III at (-6, -3) and gradually increases as x approaches positive infinity.

Answer 2

Answer:

See below

Step-by-step Step-by-step explanation:

To graph the function
`\sf y = √(x+6) - 3` and create an x and y table, we can select a range of x-values, calculate the corresponding y-values, and then plot the points on a graph.

Here's a table with some example values and the graph:
`\begin{array}c x & y \\ -2 & -1 \\ 3 & 0\\ 10 & 1 \\ \end{array}`

Now, let's plot these points on a graph:

See Attachment

The graph represents the function
`\sf y = √(x+6) - 3` and passes through the points (-2,-1), (3,0), and (10,1).

It's a curve that starts at (0, -3) and increases as x increases.