Question

Convert f(x) into vertex form, then identify the vertex.

`f(x) = -5x^(2) -40x-92`

Answer 1

Vertex form:
`\sf f(x) = -5(x + 4)^2 - 12`

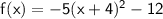
Vertex: (-4, -12)

Explanation:

To convert the quadratic function
`f(x) = -5x^2 - 40x - 92` into vertex form
`\sf f(x) = a(x - h)^2 + k`, we need to complete the square.

The vertex form of a quadratic equation allows us to easily identify the vertex.

Factor out the common coefficient (-5) from the x² and x terms:

`\sf f(x) = -5(x^2 + 8x) - 92`

To complete the square, we need to add and subtract a constant inside the parentheses such that it will be a perfect square trinomial.

To do this, take half of the coefficient of the x term
`\sf \left((8)/(2) = 4\right)`, square it
`\sf (4^2 = 16)` , and add it inside the parentheses:

`\sf f(x) = -5(x^2 + 8x + 16 - 16) - 92`

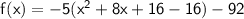
Rewrite the expression and simplify:

`\sf f(x) = -5((x^2 + 8x + 16) - 16) - 92`

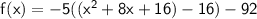
Now, we have a perfect square trinomial inside the parentheses:

`\sf f(x) = -5((x + 4)^2 - 16) - 92`

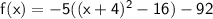
Distribute the -5 to both terms inside the parentheses:

`\sf f(x) = -5(x + 4)^2 + 80 - 92`

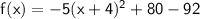
Simplify further:

`\sf f(x) = -5(x + 4)^2 - 12`

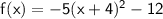
Now, the function is in vertex form
`\sf f(x) = a(x - h)^2 + k`, where the vertex is at the point (h, k).

In this case, the vertex is (-4, -12).

So, the vertex form is
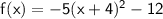`\sf f(x) = -5(x + 4)^2 - 12`, and the vertex is (-4, -12).