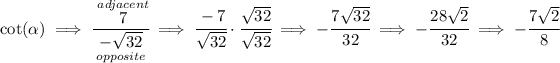Question

Please ASAP Given cos(α) = 7/9 and sin(α) < 0, calculate the exact value of cot(α).

Answer 1

Explanation:

Remember cos^2 + sin^2 = 1

(7/9)^2 + sin^2 = 1

sin^2 = 1 - (7/9)^2

sin^2 = 32/81 Take negative sqrt (because sin<0 given)

sin = - 4 sqrt 2 / 9

cot = cos / sin = 7/9 / ( - 4 sqrt 2 / 9 ) = 7/ ( - 4 sqrt 2) = - 7 sqrt 2 / 8

Answer 2

sin(α) < 0 is another way of saying sin(α) is negative, hmmm where does that happen? well, it happens only in the III and IV Quadrants, however let's notice the cos(α), has a positive 7, meaning a positive adjacent side or namely a positive cosine, hmmm so angle α is in a Quadrant with a positive cosine and a negative sine, hmmm well, that's the IV Quadrant. Now, let's find the opposite side.

`\cos(\alpha )=\cfrac{\stackrel{adjacent}{7}}{\underset{hypotenuse}{9}} \hspace{5em}\textit{let's find the opposite side} \\\\\\ \begin{array}{llll} \textit{using the pythagorean theorem} \\\\ a^2+o^2=c^2\implies o=√(c^2 - a^2) \end{array} \qquad \begin{cases} c=\stackrel{hypotenuse}{9}\\ a=\stackrel{adjacent}{7}\\ o=opposite \end{cases} \\\\\\ o=\pm√( 9^2 - 7^2)\implies o=\pm√( 81 - 49 ) \implies o=\pm√( 32 )\implies \stackrel{ \textit{IV Quadrant} }{o=-√(32)} \\\\[-0.35em] ~\dotfill`

`\cot(\alpha )\implies \cfrac{\stackrel{adjacent}{7}}{\underset{opposite}{-√(32)}}\implies \cfrac{-7}{√(32)}\cdot \cfrac{√(32)}{√(32)}\implies -\cfrac{7√(32)}{32}\implies -\cfrac{28√(2)}{32}\implies -\cfrac{7√(2)}{8}`