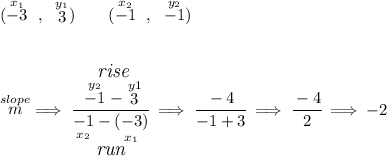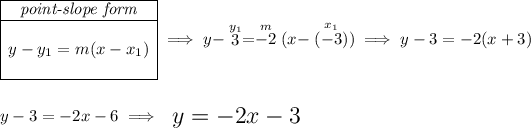Question

*PLS HELP* What is the equation for the linear model in the scatter plot obtained by choosing

the two points closest to the line?

Answer 1

now, I'm assuming the line drawn there is yours, so let me nevermind it and instead only use the points to get a best-fit line, so let's try to make a line that includes as many points as it possibly can, hmm so the line will more or less like the one in the picture below.

to get the equation of any straight line, we simply need two points off of it, let's use those two in the picture below.

`(\stackrel{x_1}{-3}~,~\stackrel{y_1}{3})\qquad (\stackrel{x_2}{-1}~,~\stackrel{y_2}{-1}) \\\\\\ \stackrel{slope}{m}\implies \cfrac{\stackrel{\textit{\large rise}} {\stackrel{y_2}{-1}-\stackrel{y1}{3}}}{\underset{\textit{\large run}} {\underset{x_2}{-1}-\underset{x_1}{(-3)}}} \implies \cfrac{ -4 }{-1 +3} \implies \cfrac{ -4 }{ 2 } \implies -2`

`\begin{array} \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{3}=\stackrel{m}{-2}(x-\stackrel{x_1}{(-3)}) \implies y -3 = -2 ( x +3) \\\\\\ y -3 = -2 x -6 \implies {\Large \begin{array}{llll} y = -2 x -3 \end{array}}`