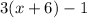Question

The linear functions f(x) and g(x) are represented on the graph, where g(x) is a transformation of f(x):A graph with two linear functions; f of x passes through 0, negative 1 and 5, 14, and g of x passes through negative 6, negative 1 and negative 1, 14.Part A: Describe two types of transformations that can be used to transform f(x) to g(x). Part B: Solve for k in each type of transformation. Part C: Write an equation for each type of transformation that can be used to transform f(x) to g(x).

Answer 1

Let's write the equation for every function:

Since f(x) passes through (0,-1) and (5,14), the equation will be given by:

`\begin{gathered} (x1,y1)=(0,-1) \\ (x2,y2)=(5,14) \\ m=(14-(-1))/(5-0)=(15)/(5) \\ m=3 \end{gathered}`

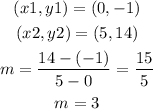
Using the point-slope equation:

`\begin{gathered} y-y1=m(x-x1) \\ y-(-1)=3(x-0) \\ y+1=3x \\ y=3x-1 \\ f(x)=3x-1 \end{gathered}`

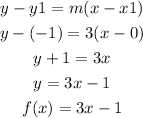
Using the same procedure for g(x):

`\begin{gathered} (x1,y1)=(-6,-1) \\ (x2,y2)=(-1,14) \\ m=(14-(-1))/(-1-(-6))=(15)/(5)^{} \\ m=3 \\ \end{gathered}`
`\begin{gathered} y-y1=m(x-x1) \\ y-(-1)=3(x-(-6)) \\ y+1=3x+18 \\ y=3x+17 \\ g(x)=3x+17 \end{gathered}`

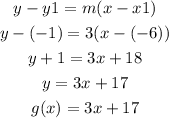
Now we have our functions:

`\begin{gathered} f(x)=3x-1 \\ g(x)=3x+17 \end{gathered}`

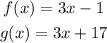
Part A:

We can translated f(x) k units up in order to get g(x) ( A vertical translation)

`f(x)=3x-1+k`

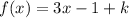
Or, we can translated f(x) k units to the left in order to get g(x) ( A horizontal translation)

`f(x)=3(x+k)-1`

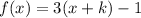
We can see a graph of the functions:

Where the red function is f(x) and the blue function is g(x).

Part B:

Since both functions must be equal:

`\begin{gathered} f(x)=g(x) \\ so\colon \\ 3x-1+k=3x+17 \end{gathered}`

Solve for k:

`\begin{gathered} k=3x+17-3x+1 \\ k=18 \end{gathered}`

-----------------------

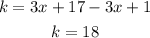
For the other case, let's use the same procedure:

`\begin{gathered} 3(x+k)-1=3x+17 \\ 3x+3k-1=3x+17 \\ 3k=3x+17-3x+1 \\ k=(18)/(3) \\ k=6 \end{gathered}`

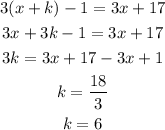
Part C:

For the vertical translation:

`3x-1+18`

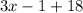
For the horizontal translation:

`3(x+6)-1`