Question

A force of 500N Is applied to a steel wire of goss_sectional area 0.2m2 the tensele stress is a. 2.5x10 1nm-2 b. 1 0x102nm-2 c. 2.5x103nw-3 d. 1.0x103nm-2

Answer 1

the tensile stress of the steel wire is 2.5 x 10³ Nm⁻².

Step-by-step explanation:

Given;

applied force, F = 5000 N

cross sectional area of the steel wire, A = 0.2 m²

The tensile stress of the steel wire, is calculated as;

`\sigma = (F)/(A) \\\\\sigma = (500)/(0.2) \\\\\sigma = 2500 \ N/m^2\\\\\sigma = 2.5 \ * \ 10^3 \ Nm^(-2)`

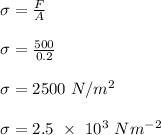
Therefore, the tensile stress of the steel wire is 2.5 x 10³ Nm⁻².