Question

What is an equation of the line that passes through the point (3, -3) and is parallel to the line x+3y=18?

Answer 1

`y=-(1)/(3)x-2`

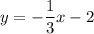
Explanation:

Linear Equations

Linear equations are typically written slope-intercept form:

y = mx + b where m is the slope and b is the y-intercept

We're given this equation: x+3y=18

Rearrange into slope-intercept form:

`x+3y=18\\3y=18-x`

`y=6-(1)/(3)x\\y=-(1)/(3)x+6`

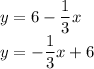
The slope of the line is
`-(1)/(3)`. A parallel line would have the same slope. Let's plug this into y = mx + b:

`y=-(1)/(3)x+b`

Now, plug in the given point:

`y=-(1)/(3)x+b\\\\-3=-(1)/(3)(3)+b\\\\-3=-1+b\\b = -2`

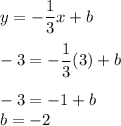
Therefore, the y-intercept is -2. Plug this back into the equation:

`y=-(1)/(3)x+b\\\\y=-(1)/(3)x-2`