Question

Triston is already 100 miles away from his home on his drive back to college. He is driving 65

mi/h.
Write a linear equation that represents d, the distance of his travel, the number of hours, h, he
driven.

Answer 1

Answer: d(h)=65h

d(hours)

rate is 65 mi/h and time is (H) Hours and in a linear equation its d(h)=65h

It relates to the distance traveled to the number of hours driven at a constant of 65 miles per hour.

Answer 2

`\sf d(h) = 65h + 100`

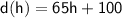
Explanation:

To write a linear equation representing the distance Triston has traveled as a function of the number of hours he has driven, we can use the formula for distance, which is given by:

`\sf distance (d)= \textsf{rate} * \textsf{time}`

In this case,

Triston is driving at a rate of 65 miles per hour, and the number of hours he has driven is represented by (h). So, the linear equation is:

`\sf d(h) = 65h + 100`

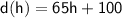
Here, d(h) represents the distance traveled after (h) hours, and 100 is added to account for the initial 100 miles he was already away from home when he started his drive back to college.